Research group: Numerics and scientific computing
Principal investigator: Professor Dr. Katharina Kormann
Our group works on the development of novel numerical simulation schemes for the solutino of partial differential equations with applications mainly in plasma physics and quantum dynamics. One focus is on structure-preserving numerical schemes that are designed to conserve certain physical invariants which renders the numerical algorithms both robust and reliable. We use compatible finite elements as well as symplectic or energy-conserving time stepping methods.
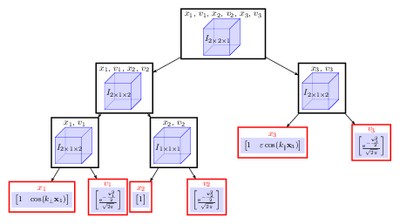
We are interested in the efficient numerical solution of high-dimensional problems and develop compression techniques and implementations for high-performance computers.
Research fields
- Algorithms and numerical analysis for high-dimensional partial differential equations
- Algorithms on high-performance computers: matrix-free algorithms, high-dimensional domain decomposition, mixed-precision, preconditioning
- Structure-preserving algorithms: discrete deRham complexes, symplectic numerical integration, structure-preserving networks
- Low-rank tensors
- Computational plasma physics